I will present some notes and explanations related to the Standard Model of particle physics and its Lagrangian . The text in this post is inspired from two answers I gave at quora.com .
The Standard model and its Lagrangian form a vast topic . I will attempt to give relevant and accurate information about it.
The story of the Standard Model started in the 1960s with the elaboration of the theory of quarks and leptons , and continued for about five decades until the discovery of the Higgs boson in 2012.
For a timeline of the history of the Standard Model see the Modern Particle Theory timeline .
The formulation of the Lagrangian of the Standard Model with its different terms and parts mirrored the theoretical and experimental advances associated with particle physics and with the Standard Model.
The Lagrangian function or Lagrangian formalism is an important tool used to depict many physical systems and used in Quantum Field Theory . It has the action principle at its basis .
In simple cases the Lagrangian essentially expresses the difference between the kinetic energy and the potential energy of a system .
The Standard Model of particle physics describes and explains the interactions between the essential components and the fundamental particles of matter , under the effect of the four fundamental forces: the electromagnetic force , the gravitational force , the strong nuclear force , and the weak (nuclear) force.
However , the Standard model is mainly a theory about three fundamental interactions , it does not fully include or explain gravitation .
The Standard model (or SM) is a gauge theory representing fundamental interactions as changes in a Lagrangian function of quantum fields. It depicts spinless , spin-(1/2) and spin-1 fields interacting with one another in a way governed by the Lagrangian which is unchanged by Lorentz transformations.
The Lagrangian density or simply Lagrangian of the Standard Model contains kinetic terms , coupling and interaction terms (electroweak and quantum chromodynamics sectors) related to the gauge symmetries of the force carriers (i.e. of the elementary and fundamental particles which carry the four fundamental interactions) , mass terms , and the Higgs mechanism term .
Explicitly , the parts forming the entire Lagrangian generally consist of :
Free fields : massive vector bosons , photons , and leptons.
Fermion fields describing matter.
The Lepton-boson interaction.
Third-order and fourth order interactions of vector bosons.
The Higgs section.
Leptons are the elementary particles not taking part in strong interactions.
All leptons are fermions. They include the electron , muon , and tauon , and the electron neutrino , muon neutrino , and tauon neutrino.
All leptons are color singlets , and all quarks are color triplets.
In the Standard model , the Higgs mechanism provides an explanation for the generation of the masses of the gauge bosons via electroweak symmetry breaking.
Different reference works , books , e-books or textbooks use different or slightly different notations and symbols to describe or designate the entities and terms within the Lagrangian of the SM .
Below is a detailed image of the Lagrangian of the Standard Model (Source: http://einstein-schrodinger.com/Standard_Model.pdf ).
However I have rearranged it and modified it with the help of Photoshop to make it look more presentable and more readable.
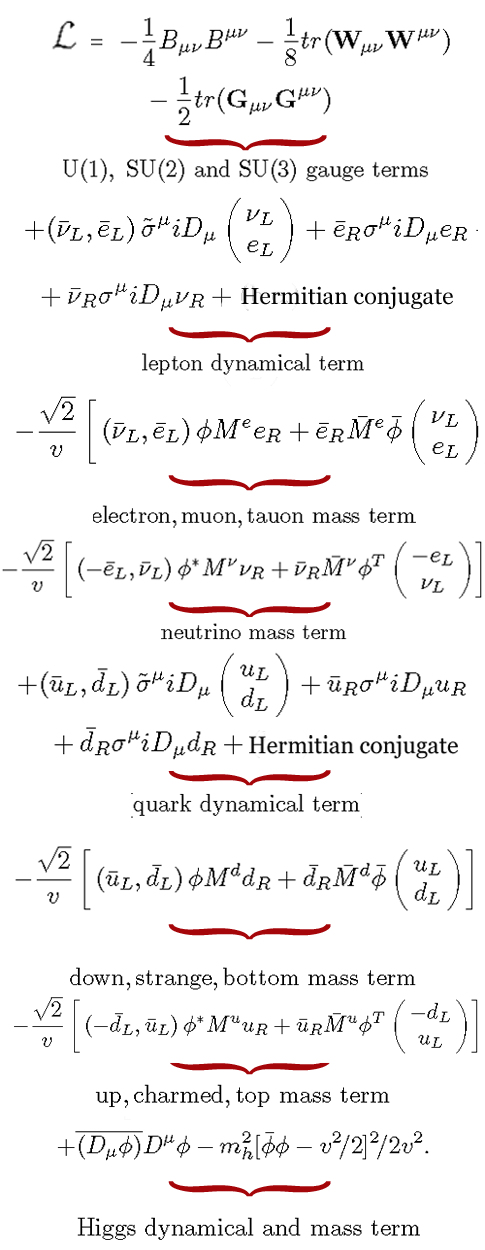
The Lagrangian function in the Standard Model , as in other gauge theories , is a function of the field variables and of their derivatives.
Gluons are the eight spin-one particles associated with SU(3).
A particle which couples to the gluons and transforms under SU(3) is called ‘colored’ or ‘carrying color’.
Gluons and quarks are confined in hadrons.
The field strength tensor
where 
The charged 
The field strength tensor
In the Standard model , electrons and the other fermions are depicted by spinor fields .
The group U(1) is the set of one-dimensional unitary complex matrices .
U(1) represents the symmetry of a circle unchanged by rotations in a plane.
SU(2) is called ‘the special unitary group of rank two’. It is a non commutative group related to SO(3) , the sphere symmetry in 3 dimensions.
SU(2) is the set of two-dimensional complex unitary matrices with unit determinant.
SU(3) , the special unitary group of rank three , is used in quantum chromodynamics (QCD) .
SU(3) is the set of three-dimensional complex unitary matrices with determinant equal to 1 .
The natural representation of SU(3) is that of 3×3 matrices acting on complex 3D vectors.
The generators of the group SU(3) are eight 3×3 , linearly independent , Hermitian , traceless matrices called the Gell-Mann matrices . These generators can be created from Pauli spin matrices (which are used with the group SU(2) ) .
The SM Lagrangian displays invariance under SU(3) gauge transformations for strong interactions , and under SU(2)xU(1) gauge transformations for electroweak interactions.
The electromagnetic group is not directly the U(1) weak hypercharge group component of the standard model gauge group. The electric charge is not one of the basic charges carried by particles under the unitary product group SU(3)xSU(2)xU(1) , it is a derived quantity.
All the masses vanish in the absence of the Lagrangian term related to the Higgs , due to the invariance of SU(3)xSU(2)xU(1) .
In some texts the gauged symmetry group of the SM is written with subscripts such as:
In the notation above , the subscript ‘c’ denotes color.
The subscript ‘L’ denotes left-handed fermions.
The subscript ‘Y ‘ distinguishes the group related to the quantum number of weak hypercharge , expressed by the letter Y , from the group associated with ordinary electric charge, expressed by Q .
The Higgs field in the Standard model is a complex scalar doublet. It is generally represented by :
In the image of the SM Lagrangian above , the Higgs field has the form
The field h(x) is real .
In the SM Lagrangian image above , 
As an additional note , the equation of the Lagrangian is usually made of a definite number of terms and Lagrangians.
In order to make such an equation look less like a big behemoth and make it more compact , it would be simpler to view it or write it first as the sum of Lagrangians :
or equivalently :
Then each Lagrangian in the equation could be expanded and explained.
Some helpful resources about the Standard Model and its Lagrangian :
Standard Model
The Standard Model of Particle Physics
Standard Model (mathematical formulation)
http://arxiv.org/pdf/hep-ph/0304186v1.pdf
Gauge Theory of Weak Interactions: Walter Greiner, Berndt Müller: 9783540878421: Amazon.com: Books
The Standard Model: A Primer: Cliff Burgess, Guy Moore: 9781107404267: Amazon.com: Books
Here is also a link to one of the important papers in the history of the Standard Model written in 1967 by Weinberg and entitled ‘A Model of Leptons’ :
http://physics.princeton.edu/~mcdonald/examples/EP/weinberg_prl_19_1264_67.pdf
Knowledge mix 